Find the Point in Which the Line Meets the Plane
Derived from the Pythagorean Theorem the distance formula is used to find the distance between two points in the plane. Let L be the line passing through the point P1 1 5 with direction vector d2 4 3T and let T be the plane defined by xy3z 1.
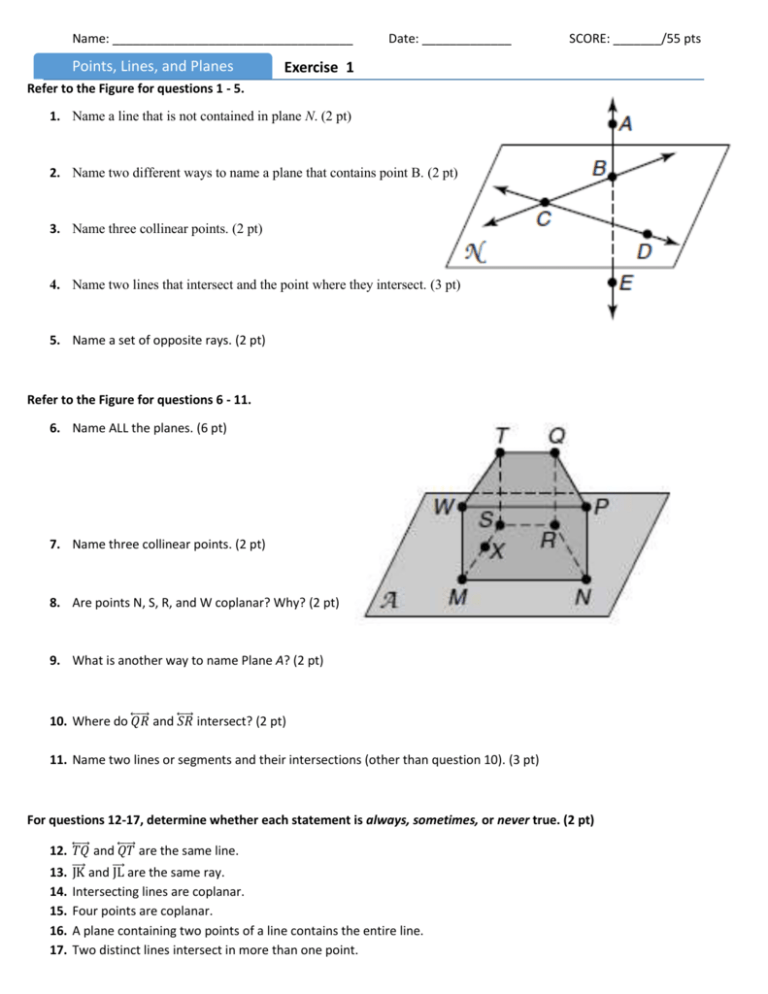
Points Lines And Planes Exercises
Plug this x y and z to.
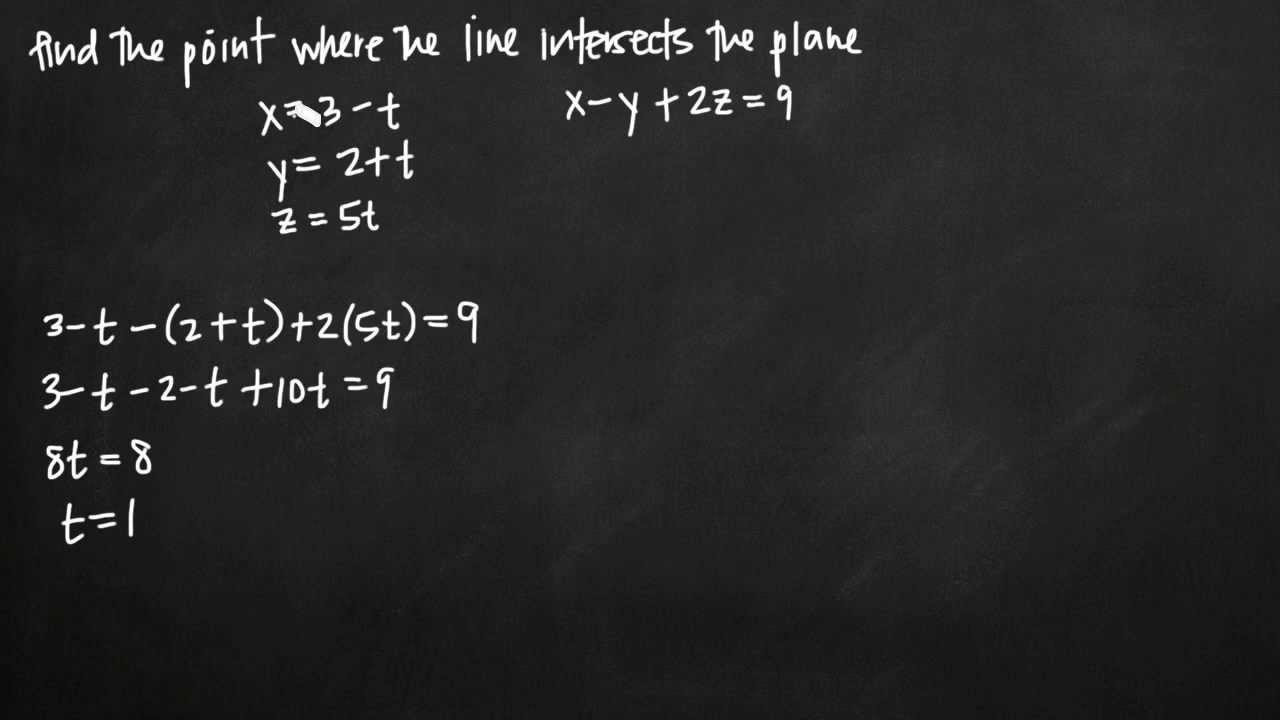
. X 3 - t y 2 t z 5t. X26t y45t z13t xyz3 The point is xyz Type an ordered triple. 2x - y 3z 6 arrow_forward Find the point in which the line through P3 2 1 normal to the plane 2x - y 2z -2 meets the plane.
Its just substitute in these equations of the line in terms of tea into the equation. The direction vector of the straight line is also a direction vector of the plane. 5 pts Find the point in which the line through the origin perpendicular to the plane 2xy z 4 meets the plane 3x5y 2z 6.
It is reasonable to expect that a line in three dimensions is given by ax by. R 1 2 3 t 0 1 3 1 to find the coordinates of the point of intersection. X 1 6t y - 2 2t z - 2 5t.
ANY point from that straight line belongs to the plane too. Plane and line intersect. 1 1 3 1 1 3 D.
X yz - 3 The point is xyz Type an ordered triple Answered. The equation of a plane is of the form Ax By Cz D. Here you can calculate the intersection of a line and a plane if it exists.
Find the point in which the line meets the plane. Find the point at which the line intersects the given plane. Solution for Find the point at which the line meets the plane.
So here were given a line in a plane and were asked to find a point where they intersect. Now putting the value of x y and z - coordinates of line in the equation of plane for finding the intersection point of line and plane we get. X t 3 y t z t 4 an equation for t.
Find the point at which the line meets the plane. Beginequationx-13 t quad y-2 quad z5 t. Answer The equation of line is x y 4 z 6 1.
There are three possibilities. Or the line could completely lie inside the plane. 125 Lines and Planes.
Quad 2 x-3 z7end. 3 1 1 3 1 1 C. The Pythagorean Theorem latexa2b2c2latex is based on a right triangle where a and b are the lengths of the legs adjacent to the right angle and c is the length of the hypotenuse.
Solve for t and use this t t 0 value in. 3 1 1 3 - 1 1 B. Example 30 Method 1 Find the coordinates of the point where the line through the points A 3 4 1 and B5 1 6 crosses the XY-planeThe equation of a line passing through two points with position vectors 𝑎 𝑏 is 𝒓 𝒂 𝜆 𝒃 𝒂 Given the line passes through the points.
Find the point Q where L and T intersect. Find the coordinates of the point where the line 2 x 1 3 y 2 4 z 3 meets the plane x y 4 z 6. X12 t quad y15 t quad z3 t.
Find the point at which the line meets the plane. Can i see some examples. The equation of a line in two dimensions is ax by c.
Find the point where the line x 1 2 y 2 3 z 3 4 x - 1 2 y - 2 - 3 z 3 4 meets the plane 2x 4y z 1 2 x 4 y - z 1. But the line could also be parallel to the plane. The line through the origin perpendicular to the plane 2x y z 4 is given by rt h000ih211it equivalently bu x 2t y t z t.
Well think about this if the plane does contain that straight line it means. This will give you a vector that is normal to the triangle. Let L be the line passing through the point P3 1 5 with direction vector d4 0 4T and let T be the plane defined by 4xy3z 16.
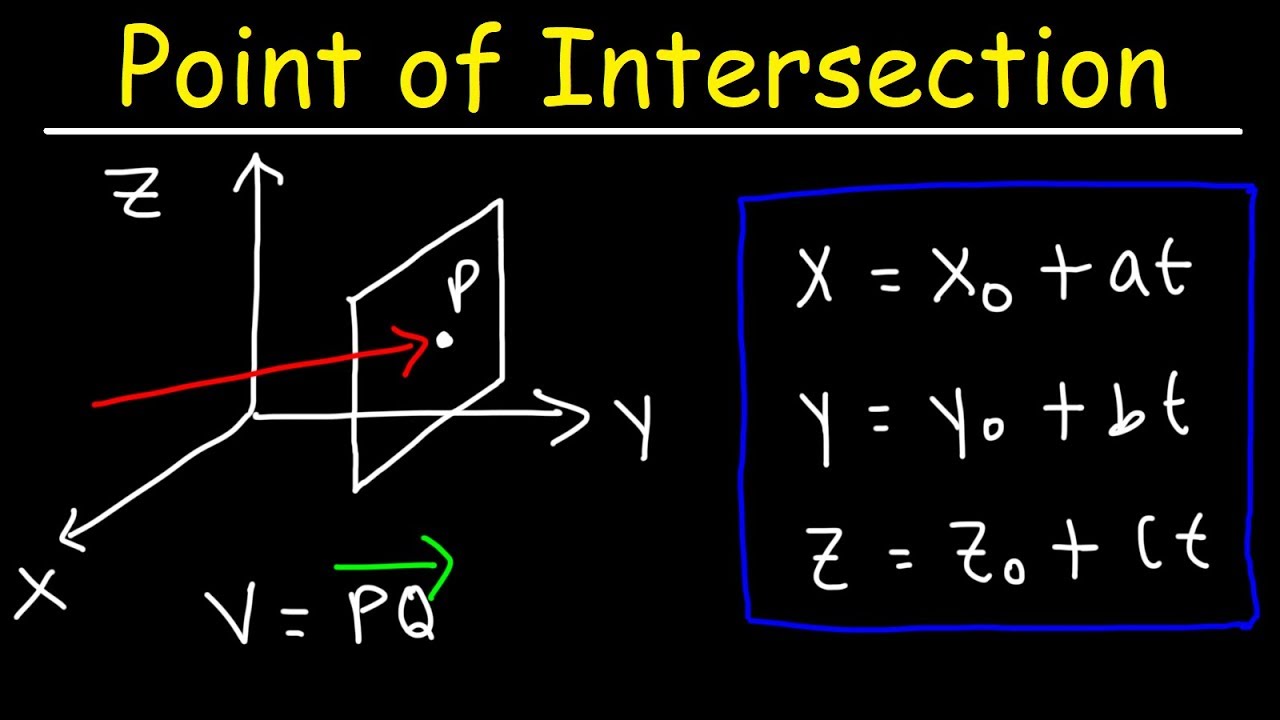
If a line and a plane intersect one another the intersection will be a single point or a line if the line lies in the plane. Enter the coordinates of points A and B and the coefficients abcd included in the equation of the plane as real numbers separated by commas as. X 1 2 y 2 3 z 3 4 x 1 2 y 2 3 z 3 4 λ Let x 2λ 1 y 3λ 2 and z 4λ 3.
X - 4 5t y 3 3t z -3 2tx y z -14 The point is x y z Type an ordered triple. X - y 2z 9. Please log in or register to add a comment.
Find the point in which the line meets the plane x 1 - t y 3t z 1 t. Find the point Q where L. This Calculus 3 video explains how to find the point where a line intersects a planeMy Website.
Z t 6 t. 1 3 1 1 3 1 class-12. Z 9 5 zfrac95 z 5 9.
The line could intersect the plane in a point. Convert the plane into an equation. To get the coefficients A B C simply find the cross product of the two vectors formed by the 3 points.
Follow this answer to receive notifications. So what we can do here. The components of this vector are coincidentally the coefficients A B and C.
Find the point of intersection of the plane r ˉ. They also will prove important as we seek to understand more complicated curves and surfaces. Putting these values together we can say the point of intersection of the line and the plane is the coordinate point.
1 1 1 2 and the line r ˉ 4 5 3 k 2 2 1 k R. Find step-by-step solutions and your answer to the following textbook question. Find the point at which the line meets bartleby.
Find the point in which the line meets the plane. Do a line and a plane always intersect. Lines and planes are perhaps the simplest of curves and surfaces in three dimensional space.
How To Find The Point Where A Line Intersects A Plane Youtube
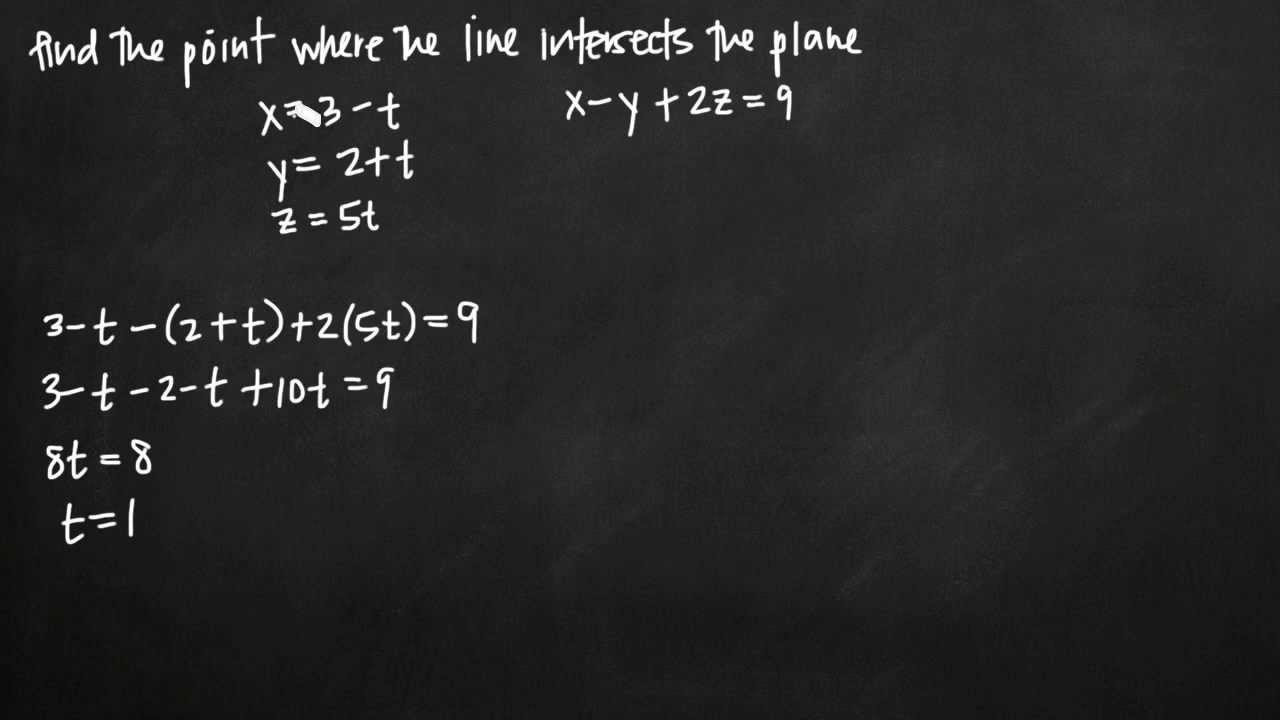
Point Of Intersection Of A Line And A Plane Kristakingmath Youtube

Finding The Intersection Of A Line And A Plane Krista King Math Online Math Help

Find The Coordinates Of A Point Where Line Intersects Xy Plane R3 Vector Youtube
No comments for "Find the Point in Which the Line Meets the Plane"
Post a Comment